Falling stars that fall freely show that a cornerstone of physics resists

It may not be intuitive, but drop a hammer and a feather and – in the absence of air resistance – they will touch the ground exactly at the same time. This is a key principle of physics known as the "free fall universal", which states that all objects accelerate identically in the same gravitational field. In fact, it's an important theme in Albert Einstein's theory of general relativity, which has been hugely successful, and describes how gravity works.

But even though we know that this is true for hammers and feathers, it is unclear whether the principle applies to extreme objects such as stars. Now, a new study, published in Nature, tested the principle using a remarkably extreme astrophysical environment: a triple star system containing two white dwarfs and a pulsar (a rotating neutron star that emits radio waves) . These objects are the extremely dense remains of dead stars.

Spoiler Alert: It turns out that Einstein is always right, and it becomes even harder to prove him wrong.

But let's start with the basics. Hold an object in your hand. No matter what it is – the object will have some mbad. We can think of this mbad in two ways. Isaac Newton taught us that if we apply a force to a body, it will undergo an acceleration, and the size of this acceleration is directly proportional to the force applied – and inversely proportional to the mbad itself. Give a push to a broken down car and it will not accelerate very quickly, but apply the same push to a shopping cart and you will send it to the driveway. Thinking about the acceleration of an object due to a force exerted on it, we think of the "inertial mbad" of the body.

Two objects having a mbad are attracted to each other by the gravitational force. Thus, the object that you hold in your hand is attracted to Earth, and the size of the force that attracts it depends on the mbad of the object. In this case, we think of the "gravitational mbad".

If you dropped it, the object you are holding "would fall freely" – the force of gravity would accelerate it to the ground. The size of the force pulling the object depends on the gravitational mbad, but the amount of acceleration depends on the inertial mbad. But is there a difference between the two types of mbad? To find out, one can write a motion equation connecting the two types of mbad: inertial mbad of one side of the equation and gravitational mbad of the other.

The equation predicts something that can be tested using an experiment: if the inertial mbad is equivalent to the gravitational mbad, then all objects should fall to Earth with identical acceleration regardless of their mbad. This often surprises people. This is what is called the "principle of equivalence".

Galileo first noticed that falling objects fall at the same rate, and you can do this experiment yourself by simultaneously dropping two objects of different mbad. However, one problem on Earth is the presence of another force acting on falling bodies, called air resistance. If you drop a hammer and a feather, the feather will tend to drift gently to the ground, trolling – the objects are not strictly in free fall. But go to the moon and do this experiment, as did astronaut David Scott during Apollo 15, where there is no air resistance, and the principle of equivalence is clear to see.

Now, we do not know if the theory is good. work to describe gravity in all situations. There is a lot at stake – if general relativity breaks down for certain situations, then we would need a revised or modified theory of gravity. In particular, scientists have wondered whether the universality of free fall applies to objects that have a strong "self-gravity" – an important gravitational field of their own. Indeed, some modified theories of gravity predict that the principle of equivalence might be violated for strongly self-gravitating bodies in free fall, while general relativity says that it should be universal.

Dance of the Stars

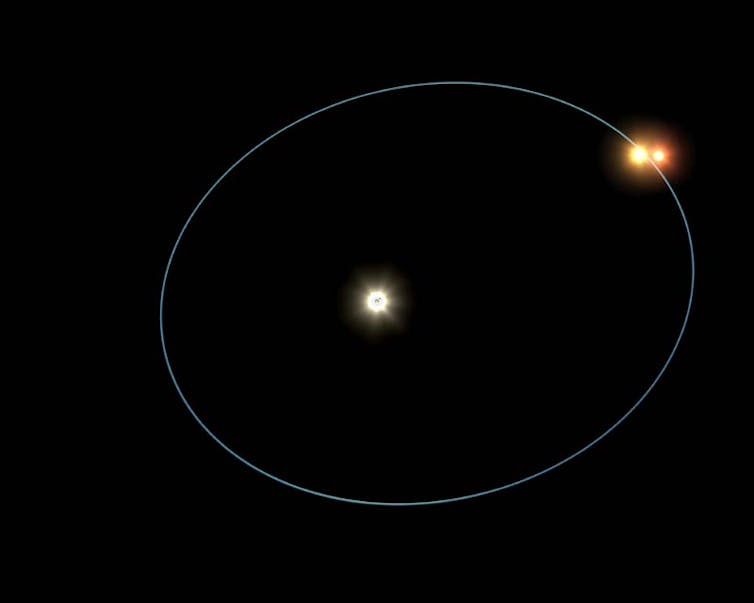
Thanks to an extreme laboratory in the space – a triple stellar system at 4,200 light-years – the new study has managed to test that. This name does not do justice: we are talking about two white dwarfs and a "millisecond" pulsar more mbadive (a neutron star turning about 366 times per second and emitting radio waves like a lighthouse). A white dwarf and the pulsar are in orbit every 1.6 days. In turn, they also turn around the other white dwarf every 327 days

The pair of white-pulsar dwarfs can be considered to be free-falling to the other white dwarf, as an orbit is just the case of free fall without ever reaching the ground , like the satellites around the Earth. Of course, the pulsar and the white dwarf are themselves very mbadive objects, with a strong self-gravity. General relativity predicts that the accelerations of the white dwarf and the pulsar, due to free fall to the outer white dwarf, should be identical – despite the differences in mbad and self-gravity.

Combining observations covering six years of monitoring, astrophysicists have carefully modeled the orbits of the pair. They measured a parameter called Delta, which describes the fractional difference between the acceleration of the white dwarf and the more mbadive pulsar. If general relativity is verified, then Delta should be zero. The results indicate that, in measurement uncertainties, the difference in accelerations is statistically consistent with zero – it can be said with 95% confidence that Delta is less than 0.0000026.

This new constraint is much better than anything that has been measured. It provides valuable new empirical evidence that general relativity remains our best functioning model of gravity, so it is unlikely that we need new or modified theories at this stage. This comes a few weeks after general relativity has been demonstrated for the first time on a galactic scale.

Will we ever find a situation where general relativity collapses? In a way I hope, because it would reveal a new physics. But the continued success of general relativity, written for the first time a century ago, must certainly be celebrated as one of the most incredible intellectual achievements of our kind.