[ad_1]
There is a mystery at the heart of our universe. A puzzle that no one has been able to solve so far. The consequences would be profound to solve it.
The mystery is why rules and mathematical diagrams seem to infiltrate almost everyone around us. In fact, there are those who describe mathematics as the underlying language of the universe.
Does this mean that it is something we have just discovered? Or is it something we invented, like any language?
We have been asking this question for thousands of years and we have not yet been able to agree.
Why the material?
Because mathematics underlies almost everything in our modern world, from computers to mobile phones to our understanding of human biology and our place in the universe.
That is why the great thinkers of history have attempted to explain the origins of the extraordinary power of mathematics.
Numbers
The modern world would not exist without mathematics. It hides behind almost everything around us and subtly influences almost everything we do now.
And yet, is invisible. I Intangible .
So where do mathematics come from?
We often think that numbers are related to objects, such as the number of fingers in a hand or the number of petals of a flower.

The flower has 5 petals. If you delete 2, there will be only 3 (and it will look less pretty).
The petals will no longer be but the number 2 will still exist.
It's something you can not say at all: if the pencils had never been invented, the idea of a pencil would not exist not.
You can destroy the physical object, burn it until only ash remains, but you can not destroy the object. idea of number
In all cultures of the world, we all agree on the concept of 4, so call it four, four four or write the symbol of another way.

The Platonic World of Numbers
Will there then be a magical world where all mathematics live? A place where are the fundamental truths that help us understand the rules of science?
Or will it be any product of our imagination and our intellect?
"It is too extraordinary to think that mathematical truths are entirely the product of our conventions in the human spirit … I do not think we are so inventive ," says Eleanor Knox, PhD in Physics Physics King's College London, UK.
"Sometimes it seems that mathematics is discovered, especially when the work is going very well and you have the impression that the equations are leading you," says Brian Greene, professor of physics and mathematics at the Columbia University, USA.
"But then you take a step backwards and realize that it is the human brain that imposes these ideas and models in the world and, from this point of view, it seems that mathematics comes from us . ", adds Greene.
"The fifth number is called fem in Swedish, my mother tongue," says Max Tegmark, a professor of physics and mathematics at MIT in the United States.
"We invented this part, the baggage, the description, the mathematical language, but the structure itself, like number 5, and the fact that it's 2 + 3, is the part we discovered, "says the Swedish expert.
The problem is that those who believe that mathematics has been discovered and those who think to invent it have very convincing arguments.
So that this series will make you change your mind again and again .
To test yourself, let's start with some simpler examples of those who say, "Mathematics is all around us, you just need to know where to look for it."
The Ingenuity of the Nautilus
![] Nautilo](https://c.files.bbci.co.uk/EA81/production/_104033006_gettyimages-548991423.jpg)
Of all the structures you find in nature, one of the most beautiful is the nautilus shell
The creature that lives inside creates all these shapes and jumps out of the way. a room on the other developing.
It is astonishing to see how this little being can create something so extraordinary and incredibly complex .
In addition, it has a hidden pattern that you can reveal by taking three pairs of measurements from the cameras.
Choose an angle and measure the inner chamber, then a second measurement to the outer edge.
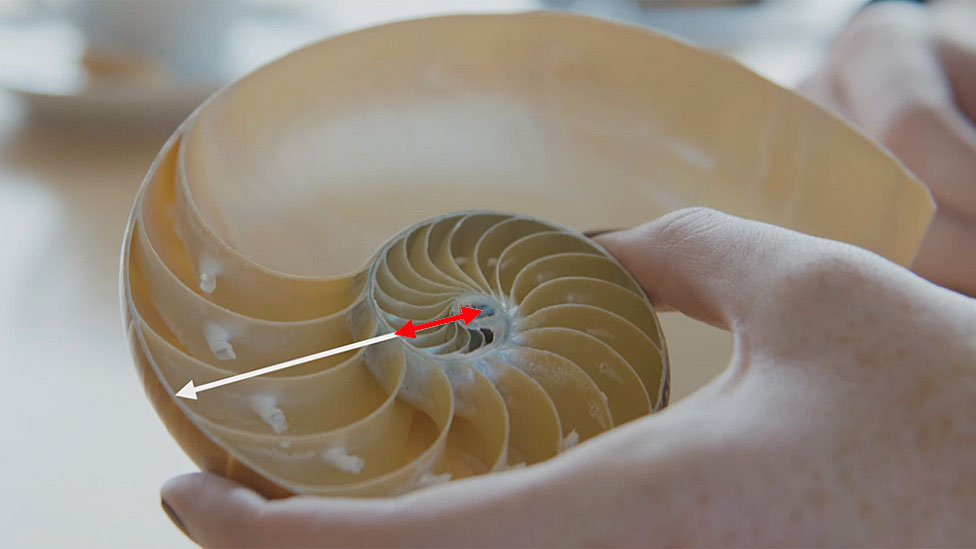
After doing this three times from three different angles, you will get three pairs of numbers that at first glance seem random.
In this case:
- 14.5 / 46.7
- 23.9 / 77.6
- 307/995
But appearances can be misleading, because if you take each of these pairs of numbers and you divide one by the other, a very clear pattern begins to appear .
- 46.7 divided 14.5 = 3.2
- 77.6 divided 23.9 = 3.2
- 995 divided 307 = 3.2
No matter where you measure the shell, the proportion The width of the cameras ends up being constant.
Whenever the nautilus makes a full turn, it ends up sitting in a chamber that is about 3.2 times the width of the previous turn.
And by repeating this simple mathematical rule, you can create this beautifully complex spiral shell. .

The petals of flowers
The nautilus is not the only one. to be alive with a mathematical pattern hidden inside.
If you have already counted the petals of a flower, you may have noticed something unusual.
Some have 3 petals. Other, 5 . Some, 8 . There are 13 petals. But they rarely have intermediate numbers (4, 6, 7, 9, 10, 11 or 12).

These figures come back again and again. They seem random, but they are all part of what is called the sequence or the succession of Fibonacci in the name of the Italian mathematician of the thirteenth century who has described in Europe.
You start with the numbers 1 and 1, then you add up the last two digits.
So …
1 + 1 = 2 ]
1 + 2 = 3
] 2 + 3 = 5
3 [5] 19659002] … and so on
Looking at the amount of petals in a flower, you discover that they follow the sequence of [Fibonacci. The same thing happens in many biological configurations, such as tree branches and leaves on stems, among others.
And that's not all.
If you look at the center of a sunflower, you will see that the seeds are arranged spirally. Count the number of spirals in one direction and you will often find a number of Fibonacci.
If you then count the spirals going in the opposite direction, you will find an adjacent Fibonacci number.
Why do plants do this? It turns out that is the best way in that the flower could organize its seeds to prevent them from spoiling.

These simple and glorious mathematical rules those who are hidden in nature do not seem a coincidence.
Once you have detected these mathematical diagrams, you feel that you have discovered them, not that you have invented them.
It's as if mathematics was waiting. you find them .
However …
For centuries, it was thought that the mathematical language was fixed and unalterable, until it became clear that something was missing: [19659015] 0 “clbad = “img-responsive” src = “https://c.files.bbci.co.uk/AE6D/production/_104035644_gettyimages-1058138808.jpg” />
What exactly is zero?
A zero means nothing. If you have zero of something, you have nothing.
0 is a strange concept; it's as if the absence was becoming something.
Is this a number or an idea? And How can something worthless have so much power ?
The 0 against The Romans

Although we have always understood the concept of To have nothing, the concept of zero is relatively new.
We used numbers, we could count but before the 7th century, zero did not exist.
The West already had a digital system: Roman numerals.
They worked well, although they were somewhat difficult to manage
It is not known if the 0 has its origin in China or India, but it is in the latter case that he was accepted as a sufficient number.
For nearly 1000 years, Indian mathematicians worked happily with Indo-Arabic numbers, while their Western counterparts kept Roman numerals until the mathematician Fibonacci recognized his potential. -Jurismi, from what I saw first-hand, how well this system of numbers worked well.
That is why he alerted Western Europe about the existence of the Indo-Arab system and defended the 0.
This new number is the one that introduced the most changes .
In 1958, for example, in Roman numerals, it is written: MCMLVIII
Wherever you were, the letter C still represents the number 100 .
0 was different . His position could change the values of the numbers around him. Think of the difference between 11 and 101 .
The 0 t e allows to write more and to manipulate them much more quickly and easily.
Now: the 0 [19659011] no what we discovered we created it as part of the language describing the numbers.
This gives the impression that mathematics is something we have imagined. We needed a more user-friendly numbering system so that someone had the brilliant idea of scratch.
It is an intriguing proof that mathematics could be invented a product of our intellect and imagination.
And this is not the only one, of course, as there are many others who support the argument that mathematics already exists and we discover it.
Q Do you think you ?
Next week: Invented or discovered? What was thought of ancient Greece of mathematics
* After the series BBC: ] "The magic numbers: the mysterious world of mathematics"
Do not forget that you can receive notifications from BBC News World. Download the new version of our application and activate it to not miss our best content.
YOU ARE INTERESTED
[ad_2]
Source link