Ultra-low gravitational field detected

Four fundamental forces are known in physics: weak and strong interactions, and electromagnetic and gravitational forces. The gravitational force is the weakest of these four. For this reason, and because experiments cannot be shielded from Earth’s gravity, measurements of the gravitational field of a test object are difficult to make in the laboratory – even for objects that have masses of several kilograms. But by writing Nature, Westphal et al.¹ report the detection of gravitational coupling between two masses of only about 90 milligrams.

Weak, strong, and electromagnetic interactions have been unified in the Standard Model of Physics, but gravitational force cannot be integrated into this model. The best model currently available to describe gravity is the General Theory of Relativity. This theory has not failed any tests so far, but there is something strange about it, as it cannot be explained in terms of quantum mechanics.

For most scientific purposes, however, we don’t need to use the general theory of relativity to explain gravity – Isaac Newton’s law of universal gravitation.² works perfectly. Published in 1687, Newton’s law states that the gravitational attraction between two bodies is proportional to their masses and inversely proportional to the square of the distance between them. This has been shown to be correct not only for describing most astronomical observations, but also in laboratory experiments. For example, the trajectory of a freely falling object (such as an apple falling from a tree) can be measured with an accuracy of less than ten parts in a billion.³, and the results are in good agreement with what one would expect from Newton’s law.

During the twentieth century, however, doubts arose about the general correctness of this law: an anomalous speed distribution of stars in galaxies was observed.⁴ in the early 1930s, and could not be explained using Newton’s law alone⁵. Even the general theory of relativity cannot account for this phenomenon. One explanation is to postulate the existence of dark matter⁶ – an invisible component, but generating gravity, of the Universe. However, no one really knows what this dark matter is made of.

Another explanation, controversial but easier to fit into models than dark matter, is that Newton’s law of gravity needs correction. A theory which attempts such a correction was proposed in the 1980s, and is called modified Newtonian dynamics.⁷. The basis of this theory is that the intensity of the gravitational field (the acceleration due to gravity) does not follow Newton’s inverse square law over large distances.

Another mystery is that the gravitational force is about 36 orders of magnitude weaker than the electromagnetic force. This is called the hierarchy problem⁸.

A framework known as string theory, which was developed in part to provide a quantum-theoretical description of gravity, addresses this problem by proposing that there are more spatial dimensions than the three we can observe. Gravity – unlike the other three fundamental forces – is believed to permeate these additional dimensions. If this is true, this could explain why the gravitational force is so much weaker than the electromagnetic force.⁹. Another consequence would be that, over a certain spatial range, the gravitational force cannot be described by Newton’s inverse square law.

Another peculiarity of gravity is that independent measurements of Newton’s gravitational constant (g, a fundamental constant used in calculations of gravitational effects) have varied widely^ten. Experimental determinations of other fundamental constants, such as Boltzmann’s constant¹¹ or the speed of light¹², converged as the number and precision of measurements increased. This has not happened since g.

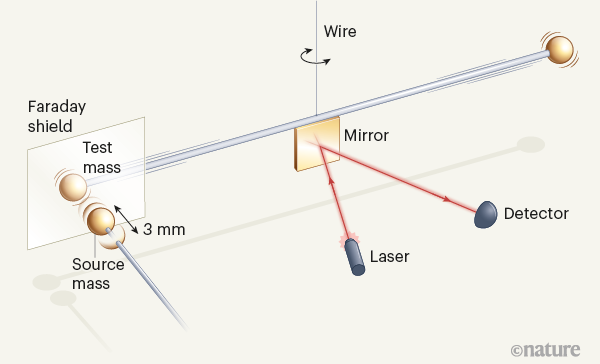
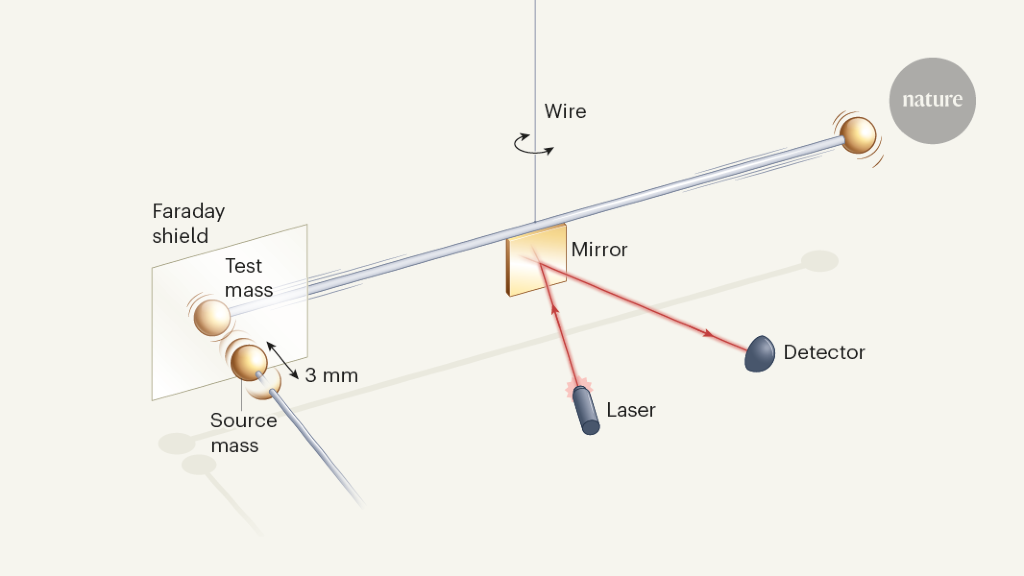
The experiments of Westphal and his colleagues could bring us closer to understanding the mysteries of gravity. They studied gravitational force using a miniature version of a torsion balancer – a device that was first used by Henry Cavendish in 1798 to measure the density of the Earth.¹³ (an experience which is equivalent to measuring g), and which is always the reference device to determine g¹⁴.

A torsion balance consists of a horizontal bar suspended in the middle from a wire suspended vertically, and with test masses attached at the ends. The gravitational attraction of the Earth acts in the vertical direction, along which the wire has great rigidity. But in the horizontal direction the wire is easily twisted and has a tiny spring constant – very small forces applied at right angles to the bar cause large rotations of the bar. The balance therefore generates an almost gravity-free environment (more precisely described as microgravity) in the horizontal plane. This is perfect for detecting small forces, such as the gravitational force exerted on the bar by a nearby object (the source mass).

In typical determinations of g, the source masses were heavy (several kilograms), to compensate for the weakness of the gravitational force. In contrast, Westphal and his colleagues used gold spheres of only 92 milligrams by mass (Fig. 1), or about the mass of 4 house flies. This is the lowest source mass ever used in such an experiment.

A calculation using Newton’s law of gravity shows that the force acting between two 90 mg spherical masses with a separation of the center of mass of 2.5 millimeters (approximately the parameters of the experiment of Westphal and his colleagues) does not is that about 9 × 10^–14 newtons. It is roughly the same force which acts on a mass of 9 picograms (1 pg is equivalent to 10^–12g) in the earth’s gravity field; to put that in perspective, 9 pg is about a third of the mass of a human red blood cell.¹⁵. The big challenge, therefore, was to extract this extremely small gravitational signal from the background “ noise ” of the experiment and from the effects of other forces (such as electromagnetic interactions) which become stronger than gravitational forces when the source and the test masses are separated by small distances.

Westphal et al. therefore modulated the signal by periodically varying the position of the source mass relative to the test mass in the torsion balance. As a result of the time-dependent gravitational interaction, the balance wheel oscillated in the horizontal plane at the frequency of the signal modulation (12.7 millihertz). Because the gravitational field of a spherical mass is nonlinear (a consequence of Newton’s inverse square law), the balance is also stimulated to oscillate at higher frequencies that are multiples of the modulating frequency (called harmonics). higher). This effect could be clearly identified in the experiment, thus providing a signature of the gravitational coupling between the masses.

The detection of such a tiny gravitational signal is in itself an exciting result, but the authors went even further by determining a value for g of their experience. Their estimate deviates from the internationally agreed value (see go.nature.com/2bwkrqz) by about 9% – a small amount, since the experimental uncertainties of their system have not yet been optimized for precise measurements of g. The experiment is therefore the first to show that Newton’s law of gravity is valid even for source masses as small as these.

The next step is to reach even smaller masses – Westphal et al. suggest that gravitational fields of masses of the order of 10^–8kg could possibly be measured. However, there is still a long way to go to achieve this goal. The first task will be to significantly reduce the damping of the oscillations of the torsion balance, which will not be easy. But if it can be done, then maybe quantum gravitational effects will eventually be observed.